Trigonometric Functions in a Right Triangle

In this section, we introduce the trigonometric functions using a right triangle. There are 6 main trigonometric functions: sine, cosine, tangent, cotangent, secant, and cosecant. For acute angles, these functions can be defined as ratios between the sides of a right triangle.

Consider a right triangle ABC with an acute angle A = α. The side b between the angle α and the right angle C is called the adjacent leg to angle α. Respectively, the other leg a is called opposite to angle α.

Trigonometry in a right triangle — Figure 1.

The sine of \(\alpha,\) abbreviated \(\sin \alpha,\) is the ratio of the length of the opposite leg to the length of the hypotenuse:

\[\sin \alpha = \frac{a}{c} = \frac{\text{opposite leg}}{\text{hypotenuse}}\]

The cosine of \(\alpha,\) abbreviated \(\cos \alpha,\) is the ratio of the length of the adjacent leg to the length of the hypotenuse:

\[\cos \alpha = \frac{b}{c} = \frac{\text{adjacent leg}}{\text{hypotenuse}}\]

The tangent of \(\alpha,\) abbreviated \(\tan \alpha,\) is the ratio of the length of the opposite leg to the length of the adjacent leg:

\[\tan \alpha = \frac{a}{b} = \frac{\text{opposite leg}}{\text{adjacent leg}}\]

The tangent function can be expressed in terms of the sine and cosine:

\[\tan \alpha = \frac{a}{b} = \frac{a}{c} \cdot \frac{c}{b} = \frac{{\frac{a}{c}}}{{\frac{b}{c}}} = \frac{{\sin \alpha }}{{\cos \alpha }}.\]

If we take the reciprocals of the sine, cosine, and tangent functions, we obtain expressions for the other \(3\) trigonometric functions.

The reciprocal of the sine function is called the cosecant. The cosecant of \(\alpha,\) denoted \(\csc \alpha,\) is the ratio of the length of the hypotenuse to the length of the opposite leg:

\[\csc \alpha = \frac{1}{\sin\alpha} = \frac{c}{a} = \frac{\text{hypotenuse}}{\text{opposite leg}}\]

The reciprocal of the cosine function is called the secant. The secant of \(\alpha,\) denoted \(\sec \alpha,\) is the ratio of the length of the hypotenuse to the length of the adjacent leg:

\[\sec \alpha = \frac{1}{\cos\alpha} = \frac{c}{b} = \frac{\text{hypotenuse}}{\text{adjacent leg}}\]

The reciprocal of the tangent is called the cotangent. The cotangent of \(\alpha,\) denoted \(\cot \alpha,\) is the ratio of the length of the adjacent leg to the length of the opposite leg:

\[\cot \alpha = \frac{1}{\tan\alpha} = \frac{b}{a} = \frac{\text{adjacent leg}}{\text{opposite leg}}\]

Example

The top of the Eiffel Tower is seen from a distance of \(d=500\,m\) at an angle of \(\alpha = 31^\circ.\) Find the tower's height.

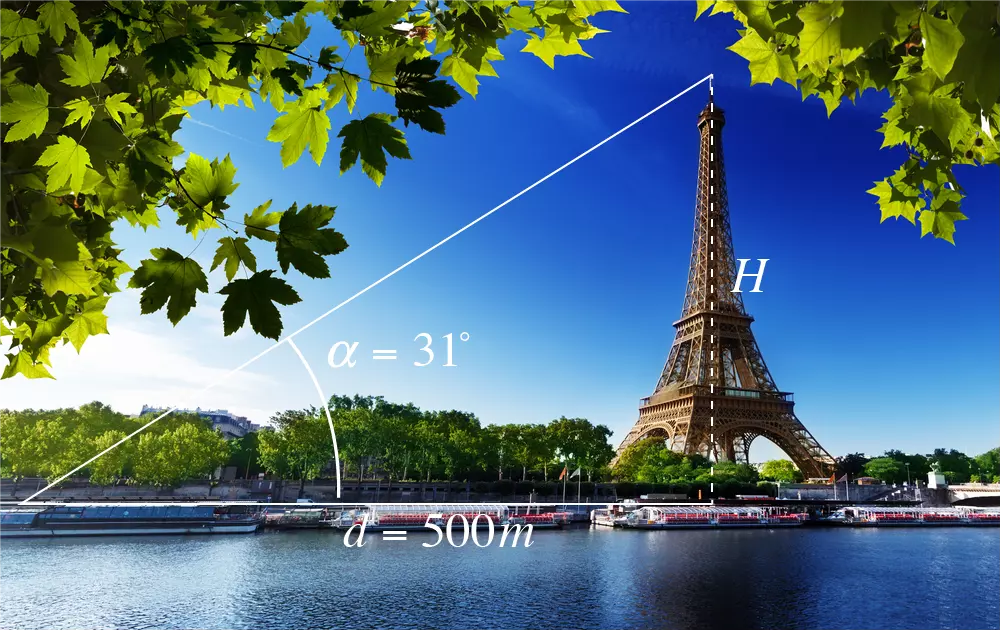
Finding the height of Eiffel Tower. — Figure 2.

Solution.

We know the angle of elevation \(\alpha\) and the adjacent leg \(d.\) The tower's height \(H\) is the opposite leg in the right triangle. Hence,

\[H = d\tan \alpha = 500 \times \tan {31^\circ} = 500 \times 0,6 = 300\,m.\]

See solved problems on Page 2.